Disaggregation of "Elhuyar", "Elhuyaw", ": Semiordenak
Analisi Matematikoa saileko katedraduna eta Matematika mintegiko burua
Nafarroako Unibertsitate Publikoa
Askatasuna BHI institutuko Matematika irakaslea eta NUPeko Analisi Matematikoa sailean irakasle laguna

If in an Internet search engine we wrote "Elhuyaw" (sic), then we would get the message "You wanted to write Elhuyar" and then the references to "Elhuyar" would appear before our eyes. On this occasion, and with the same objective, if we wrote "Elhuyww", the search engine would respond immediately with the message "No results have been found". The words "Elhuyaw" and "Elhuyar" differ by a letter, while the difference between "Elhuyar" and "Elhuy2-2" may be due to a different response.
Perhaps the search engine has a specific program to detect the difference of a letter between two words, so if we write "Elhuyaw" it has realized that there has been an error and has supposed that we wanted to write "Elhuyar". Similarly, the search engine is not able to assume that once written "Elhuy2-W" it is an error due to the difference of two letters.
It can happen that the search engine has a threshold of perception (Threshold of perception in Spanish; threshold perception in English), that is to say, if in a letter they differentiate as maximum two words, reads the programs as inseparable and emits the message "Maybe ...", but if we write two or more letters wrong, understand that we are looking for something different: Elhuyar and Elhuyar are different and their results. Let us see that this relationship of inseparability between the words of the example is not transitory: Elhuyar and Elhuyar are inseparable (because they differ in a letter). For the same reason, "Elhuyaw" and "Elhuyff" are inseparable, but differentiate the words "Elhuyar" and "Elhuyww", and thus acts the computer.
With this introduction, the members of the research group on "Mathematics of the Order" (UPNA, Pamplona), with the grant of the research project MTM2007-62499, want to launch a small section in which we are working in recent years: comparisons between options or alternatives, non-transitory indexes and thresholds of perception, basic concept of our latest research, called semiorder. Among its applications is the configuration of search programs in the network browsers.
Taking into account the historical principle, this concept is N. It appears implicitly in a work by Wiener of 1914. Years later, in 1956, R. D. D. Luce used it in the economic field (ber) to analyze situations of comparison of opportunities or alternatives and to make decisions in which agents participate who must establish priorities in situations of non-transitory indifference. R. R. D. D. The name of semiorder (semiorder, in English; quasi-ordre francés) corresponds to the author Luce...
Priority systems and semiorders
When analyzing a set of options, two relationships between different options are detected, if we have two options, one more suitable, preferred or desirable. But they can also be similar. In this case, it gives us the same one or the other, because we will not separate it. We will study the properties of relationships "being more appropriate" and "being inseparable" to obtain the definition of the structure of semiorders. Being X a set of options or alternatives and P, if it is two binary relations defined in this group I, it is understood that the pair of relationships [P, I] is a priority system: The P relationship will be used to express specific priorities (more appropriate) and the I relationship to express inseparability.
That is, if x and y X are two options or alternatives and we like more than x option and (we write x P and), by chance it can not be and more than x (if x P and it is impossible and P x ), so P must be asymmetric. Options x and y may be the same or the alternatives are the same, but we will overcome this problem with unthinking P (if x is an alternative, x P x). On the other hand, the relationship I must be reflective, that is, if x I x ; x is an option, it cannot be differentiated. In addition, we will define relationship I when we have a relationship P, not the other way around. With this we want to indicate that two options will be inseparable and we will write x I and if it is not x P and neither P x. Another property of I is that it is symmetric (if x I and, and I x).
We are currently able to define the concept of semi-order, so we will not use R. D. D. He who used Luce, who is completely technical: Aleskerov et al. We will use the provisions of chapter 3.2 of the work (2007).
If the priority system is defined in group X [P, I], it will be considered that the specific priority ratio P is a semiorder, x; y; z; any element of the set t X meets two conditions:
1. If x P and ; and I z ; z P is filled, you should fill x P t. (PIP B)
2. If x P and ; and P z ; z I was filled, you should fill x P t. (PPI B)
Thresholds of perception
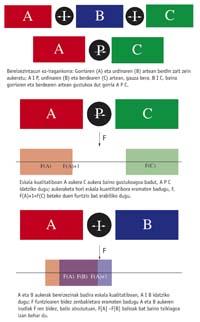
In the definition of semiorders the threshold of perception does not appear directly, but non-transitory indifference shows us that it is necessary, in the introductory example "Elhuyar" I "Elhuyaw" I "Elhuyww", but we have seen that "Elhuyar" is "P "Elhuyar", and we have assumed that if the number of different letters is greater than one separate. We will work the constant border between what we can choose and what they are inseparable. Although Luce took into account the idea in his original article, it was Scott and Suppes, in a 1958 paper, who analyzed the concept directly. In this work a very important result of the semiorders defined in finite groups was demonstrated:
Scott-Suppes Theorem [1958]: Since a finite set X and the P ratio are a semi-order defined in the set X, there is then a function F defined in the set X and which takes real values, where x and are two alternatives, x is more satisfied than and only if it is fulfilled that F(x)+1 < F(y).
If we look at the meaning of this basic theorem, we will observe that the semiorder P, based on the comparisons between the different options, and which is defined in most of the occasions that we select the preferences of the options on a quantitative scale, passes on a qualitative or numerical scale. That is to say, each of the x options of group X, through function F, corresponds the number F( x ), (and to F( and ), so that, comparing the real numbers F( x ) and F( and ), we can know if we like more than the alternative and x. This P priority is determined by a constant perception threshold: Scott-Suppes Teoreman The perception threshold is a number. We will note that x is more desirable than and only if the perception threshold F( and ) - F( x ) is greater than one. However, if in absolute value F( and ) - F( x ) is less than one, then the x and y options are inseparable.
To work the Mathematics of the Order, it is necessary to have this type of translations in quantitative scales equivalent to types of ordination in qualitative scales, such as the semiorders. That is, to know more and less among the numbers that is habitual through the relation and not qualitatively the options defined in a set (defined by a binary relationship or by an arrangement). The Scott-Suppes Theorem in a finite set transforms the scales of semiorder into numerical scales through function F and a threshold of constant perception.
The problem of numerical expression
The result we have released on the portal (Scott-Suppes Theorem), which states that a semiorder called P in finite sets X can be representative by a function F and a threshold of perception, is not fulfilled in infinite sets. Therefore, we will say that a semi-order P defined in an infinite set is representative in the form of Scott-Suppes if we can represent it by a function F and a threshold of perception: When function F is defined in group X and takes real values and we have two alternatives x e and y is x P and only if F( x ) + 1 < F( and ).
Now, in this general situation, there are semi-orders P defined in a set X (necessarily with infinite elements) that do not support this type of expression. For them it is not possible to find an expression like Scott-Suppes, an adequate F function and a constant perception threshold. Examples such as: In a group X we have a sequence x ( n ) in which each element is more satisfactory than the following (x ( n) P x ( n +1) n for all natural numbers) and an element x * for all subjects that meet x ( n) P x * (all consecutive elements are more satisfied than it), in this situation it is impossible to give an expression in the form of Scott-Suppes. Abrisqueta et al. In (2009) different examples appear.
The time has come to raise the key to this theory. The problem of the numerical expression of the semiorders: What are the characteristics of a semiorder P defined in a set X for this semiorder P to be representative in the form of Scott-Suppes? We have been studying this problem for fifteen years in some research groups. Although we have obtained some of the general results, it is very technical to explain here. For more information on this topic is interesting Abrisqueta et al. (2009) Reference article, in which the details of what was carried out in relation to this problem are analyzed.
In general, as mentioned above, the attainment of numerical expressions of ordered structures is the headache of an active branch of the Mathematics of the Order, namely, the attainment of numerical expressions of ordered structures. We can make a qualitative scale understood through a quantitative scale. Among these structures, the semi-orders have undoubtedly been the most difficult problem since Scott-Suppes presented his work. The leap of qualitative scale to quantitative scale in other ordered structures has been given for several years. The responses obtained in the semiorders require a high degree of abstraction of difficult development. Perhaps we should simplify them, trying to find alternative answers more simple than those we currently have. In that we are.





